Instructional Next Steps 

 
The CenterPoint Interim Assessments are meant to provide information about student progress towards end-of-year expectations and to identify areas of strength and improvement regarding how well students can solve problems in mathematics aligned to college- and career-ready standards. Additionally, the questions on the interim assessments are like those on state summative assessments, thus, providing an indication of student progress throughout the year and a better understanding of what to expect on end-of-year assessments.  

The score reports from the interims provide data that can be used to deepen educators’ understanding of their students’ learning progress toward college and career readiness and to determine patterns of student performance to diagnose students’ strengths and areas of need.  
 

Interim Expectations 

 
In mathematics, students meet expectations when they can:  

• solve problems involving the Major Content of the grade/course with connections to the Standards for Mathematical Practice; 
• solve problems involving the Additional and Supporting Content of the grade/course with connections to the Standards for Mathematical Practice; 
• express grade-/course-level-appropriate mathematical reasoning by constructing viable arguments, critiquing the reasoning of others, and/or attending to precision when making mathematical statements; 
• solve real-world problems with a degree of difficulty appropriate to the grade/course by applying knowledge and skills articulated in the standards for the current grade/course, engaging particularly in the Modeling practice, and, where helpful, making sense of problems and persevering to solve them (Mathematical Practice 1), reasoning abstractly and quantitatively (MP.2), using appropriate tools strategically (MP.5), looking for and making use of structure (MP.7), and/or looking for and expressing regularity in repeated reasoning (MP.8). 

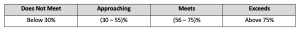
When considering a student’s score on the interim assessments, consider the achievement levels in the table. As an example, a student that scores 70% is likely meeting expectations.  

Of note: CenterPoint’s interim assessments are designed to show students’ progress toward meeting end-of-year expectations. Be cautious when reviewing student data at the standards level so as not to jump to immediate conclusions since most standards are assessed with a minimal number of questions. This design was intentional to keep testing time to a minimum, ensure assessment coverage of the standards, and provide a high-level view of progress towards meeting end-of-year expectations. Also, the subclaim data provides a more complete picture of student proficiency when looking at all the data points from the three assessments completed within the year. 
 

Strategies for Supporting Students in Mathematics 

 
When students have not yet earned scores that indicate on-track performances, the following instructional supports may be provided: 

• Use the applicable additional resources within the curriculum to support students in areas of need.  
• Consider how the curriculum allows for embedding additional supports within upcoming lessons, thereby allowing for supports to be provided as part of daily core general instruction. Work with school leaders to think creatively about structures that allow additional time for students to strengthen their math skills and understandings, especially for those needing intensive support. 
• Have students describe their thinking as they solve math problems. This can be done using questions from the interim, released items from state summative assessments, or other open resources that are well known for producing quality content. A list of resources at the end of this document may be of help. Since students may be unfamiliar with how to think aloud, teachers will likely want to model the process with a sample question. The act of listening to students as they think aloud is a great means to help teachers and students uncover conceptual misunderstandings and provide insight into the nature of erroneous thinking. 
• Review student’s scratch paper from the interims to investigate misunderstandings and errors. 
• Teachers in a professional learning community (whether formal or informal) may find it helpful to share ideas on how to support students who are struggling with mathematics at a given grade level.  
• Model multiple techniques and approaches to demonstrate different pathways to solving problems. 
• Use mathematics manipulatives to help students conceptualize abstract concepts. 
• Have students work with others to solve problems. 
• Create scaffolded problem sets to chunk learning. 
• Create centers targeted to support areas of need. 
• Use easier numbers in problem sets to uncover conceptual misunderstandings. 
• Share sample math questions with resource teachers and those who teach content other than mathematics but whose content areas apply mathematics (e.g., science, computer science, technology education, etc.), and share how they can support students who require additional instruction and practice for identified skill gaps. 

Math Resources for Students Needing Support 

 
First, consider utilizing the resources that come with the curriculum when considering how to provide support for students. Then, the list below may be of help. 

• Illustrative Mathematics: free access to their library of mathematics curriculum, instructional tasks, and resources, including math resources for families. 
• Student Achievement Partners: high-quality, open-source classroom resources, including math lessons and assessments.  
• Khan Academy: free standards-aligned lessons and practice in Math, Science & Engineering, Arts & Humanities, and the SAT. 
• Citizen Math: Search by standard or math topic to find math tasks related to real-world challenges. The Citizen Math mission is to make the world a better place by inspiring young people to develop the problem-solving skills they will need to analyze, discuss, and solve the important issues faced by society. 

Prev        Next